Solve the equation f(x) = 0 analytically and then use the graph of y = f(x) to solve the inequalities f(x) < 0 and 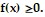 f(x) = 4 - 2 log4(x + 8)
f(x) = 4 - 2 log4(x + 8)
A. {248}; (248, ?); (-8, 248]
B. {8}; (-8, 8); [8, ?)
C. {8}; (8, ?); (-8, 8]
D. {8}; (8, ?); (-?, 8]
Answer: C
You might also like to view...
Solve by the quadratic formula. Simplify answers. Use i notation for nonreal complex numbers.x2 + 8x + 25 = 0
A. x = -4 ± 9i B. x = -4 + 3i C. x = -7, -1 D. x = -4 ± 3i
Simplify the expression. Assume that all variables are positive when they appear.-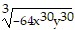
A. 4x10y10 B. -4x30y10 C. 16x10y10 D. 4x10y15
Find the real or imaginary solutions by using the quadratic formula.x2 - 8x + 20 = 0
A. {-4 ± 2i} B. {4 ± 2i} C. {6, 2} D. {8 ± 4i}
Find vectors b and c and matrix A so that the problem is set up as a canonical linear programming problem of the form: maximize cTx subject to Ax ? b and x ? 0. Do not find the solution.Maximize 5x1 + x2 + 6x3 subject to x1 + 4x2 - 4x3 ? 34 -4x2 + 4x3 ? 20and x1 ? 0, x2 ? 0, x3 ? 0
A. b = 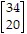 , c =
, c = 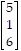 , and A =
, and A = 
B. b = 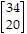 , c =
, c = 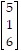 , and A =
, and A = 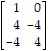
C. b = 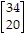 , c =
, c =  , and A =
, and A = 
D. b = 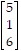 , c =
, c = 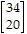 , and A =
, and A = 