Answer the question or provide the appropriate response.Explain how you would solve the following problem graphically. (Do not actually solve the problem.) If a rectangle has perimeter 74 inches, what is the maximum area that it can have? (Let x represent the width of the rectangle.)
A. Graph the function y = -x2 + 37x and find its x-intercepts. The larger x-intercept gives the maximum area (in square inches).
B. Graph the function y = -x2 + 37x and find its vertex. The x-coordinate of the vertex gives the maximum area (in square inches).
C. Graph the function y = -x2 + 37x and find its vertex. The y-coordinate of the vertex gives the maximum area (in square inches).
D. Graph the function y = -x2 + 74x and find its vertex. The y-coordinate of the vertex gives the maximum area (in square inches).
Answer: C
You might also like to view...
Fill in the blank with <, >, or = to make a true comparison statement. 0.5  0.4
0.4
A. = B. < C. >
Divide, if possible. If a quotient is undefined, state so.
A. -1 B. -2 C. 2 D. -3
Decide whether or not the transition matrix is regular.
A. Yes B. No
Graph the parabola.y = -5(x - 1)2 - 5
A. 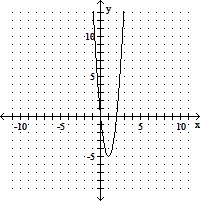
B. 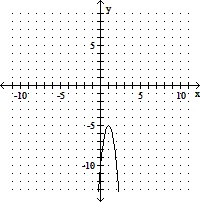
C. 
D.