Provide an appropriate response.Prove that loga(M/N) = loga(M) - loga(N). Hint: Recognize that M = ax and N = ay.
What will be an ideal response?
Answers will vary. One possibility is:
Using the properties of exponents:
M/N = 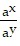 = axa-y = ax-y.
= axa-y = ax-y.
By the definition of logarithm, M/N = ax-y is equivalent to
loga(M/N) = x - y.
Since M = ax, then x = loga(M), and since N = ay, then y = loga(N). Substituting, we get
loga(M/N) = loga(M) - loga(N).
You might also like to view...
Use the at least once rule to find the indicated probability.Find the probability of at least one 3 in 7 rolls of a fair die.
A. 0.721 B. 0.992 C. 0.279 D. 0.056
Answer the question.A bag of pecans holds at least 45 nuts and at most 65 nuts. How many bags of pecans must be selected to be sure that at least three bags contain the same number of nuts?
A. 44 bags B. 43 bags C. 23 bags D. 22 bags
Solve the problem.How many cubes with 1-inch sides can be placed in a rectangular solid of length = 6 inches, 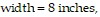 and
and 
A. 56 B. 336 C. 21 D. 42
Solve.A regular pentagon has a side length of 2.4 mi. Find its perimeter.
A. 14.4 mi B. 12 mi C. 9.6 mi D. not enough information given