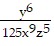Solve the problem.The economy of a small country can be regarded as consisting of three industries, I, II, and III, whose input-output matrix isIIIIII 
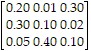Suppose x, y, and z represent the output of industries I, II, and III, respectively. An algebraic expression for the amount of output from industry III that can be exported is
A. z + (0.05x + 0.40y + 0.10z).
B. z + ( 0.30x + 0.02y + 0.10z).
C. z - (0.05x + 0.40y + 0.10z).
D. z - (0.30x + 0.02y + 0.10z).
E. none of these
Answer: C
You might also like to view...
Factor out the greatest common factor.t(2 - m) + s(2 - m)
A. (t + s)(2 - m) B. (t - s)(2 - m) C. No common factor (except 1) D. t(2 - m) + s
If f satisfies the equation of change 
, then what can be said about the function 
?
?
A. f is linear with slope 4.
B. f is linear with slope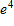 
.
C. f is exponential with growth factor 4.
D. f is exponential with growth factor 
.
Describe all symmetries of the figure shown.
A. 2 lines of symmetry, 180° rotation symmetry B. 1 line of symmetry, 180° rotation symmetry C. 4 lines of symmetry, 90° rotation symmetry D. 2 lines of symmetry, 90° rotation symmetry
Simplify the exponential expression.(5x-6y3z-2)-3
A.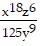 
B.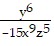 
C. 
D. 