Solve the problem.A population grows according to the logistic growth model, with growth parameter r = 1.6 and initial population given by p0 = 0.5. What does the logistic growth model predict in the long term for this population?
A. The population settles into a four-period cycle with the following approximate percentages of the habitat's carrying capacity: 50%, 37.9%, 38.4%, 37.5%.
B. unlimited growth
C. The population settles into a two-period cycle alternating between a high-population period at 50% and a low-population period at 37.5% of the habitat's carrying capacity.
D. extinction
E. It stabilizes at 37.5% of the habitat's carrying capacity.
Answer: E
You might also like to view...
Find the indicated root of the given quadratic equation by finding x3 from Newton's method (choose x0 to be the midpoint of the given interval).2x2 - 2x - 3 = 0 (between 1 and 2)
A. 1.8274221 B. 1.8228757 C. 1.8274457 D. 1.8228760
Solve the problem.Find all values of k so that the given points are 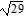 units apart. (-5, 5), (k, 0)
units apart. (-5, 5), (k, 0)
A. 3, 7 B. 7 C. -3, -7 D. -7
Answer the question or give an explanation.Describe in your own words how to reconcile a checking account.
What will be an ideal response?
Solve the inequality and write the solution set in interval notation.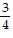 t ? - 60
t ? - 60
A. (-?, 80] B. [80, ?) C. (-?, -80] D. [-80, ?)