Find the vertex of the parabola and determine if it is a maximum or minimum. y = 6x2 + 3
A. (0, - 3); minimum
B. (0, 3); maximum
C. (0, 3); minimum
D. (0, - 3); maximum
Answer: C
You might also like to view...
Solve using the zero-factor theorem.b(b + 14) = 0
A. -14, 0 B. 14, 0 C. -1, -14 D. 1, -14
Sketch the graph of the equation. Identify the vertex and the intercepts.y = x2 + 5x + 4
A. vertex:  ;
;
x-intercepts: (-1, 0) and (-4, 0);
y-intercept: (0, 4)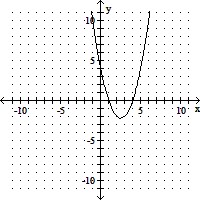
B. vertex: 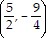 ;
;
x-intercepts: (1, 0) and (4, 0);
y-intercept: (0, 4)
C. vertex:  ;
;
x-intercepts: (1, 0) and (4, 0);
y-intercept: (0, 4)
D. vertex: 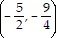 ;
;
x-intercepts: (-1, 0) and (-4, 0);
y-intercept: (0, 4)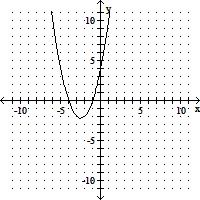
Solve the problem.Find (fg)(x) given f(x) = 3x + 1 and g(x) = 5x2 - 1.
A. 15x3 - 1 B. 3x3 + 5x2 - 1 C. 15x3 + 5x2 - 3x - 1 D. 15x3 - 3x2 + 5x - 1
Find the intervals on which the function is increasing and the intervals on which the function is decreasing.f(x) = x2 - 12x - 28
A. Increasing on (-?, 6); decreasing on (6, ?) B. Increasing on (- 6, ?); decreasing on (-?, - 6) C. Increasing on (6, ?); decreasing on (-?, 6) D. Increasing on (-?, - 6); decreasing on (- 6, ?)