Subtract.12,141
A. 4,166
B. 4,164
C. 11,166
D. 4,084
Answer: A
You might also like to view...
Solve the application problem.Jim can run 5 miles per hour on level ground on a still day. One windy day, he runs 13 miles with the wind, and in the same amount of time runs 6 miles against the wind. What is the rate of the wind? (Round your answer to the nearest tenth, if necessary.)
A. 3.5 mph B. 13.6 mph C. 5 mph D. 1.8 mph
Find the linearization of the function at the given point.
at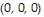 
A.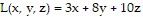 
B. L(x, y, z) =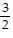 
x + 4y + 5z
C. L(x, y, z) =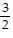 
x + 4y + 5z + 1
D.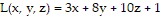 
Solve the problem.Ken is 6 feet tall and is walking away from a streetlight. The streetlight has its light bulb 14 feet above the ground, and Ken is walking at the rate of 3.3 feet per second. Find a function, d(t), which gives the distance Ken is from the streetlight in terms of time. Find a function,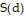 
, which gives the length of Ken's shadow in terms of d. Then find 
.
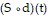A. (S ? d)(t) = 5.58t B. (S ? d)(t) = 2.48t C. (S ? d)(t) = 1.82t D. (S ? d)(t) = 3.14t
Solve the problem.Find two functions defined by f(x) and g(x) with the following properties: 
A. f(x) = x2; g(x) = x B. No such functions exist. C. f(x) = 1; g(x) = x D. f(x) = x; g(x) = x2