Begin by graphing the standard cubic function f(x) = x3. Then use transformations of this graph to graph the given function.h(x) = (x + 3)3 + 4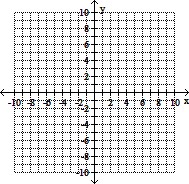
A. 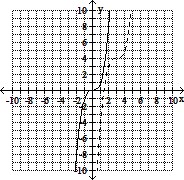
B. 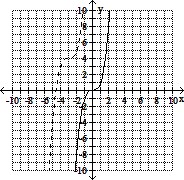
C. 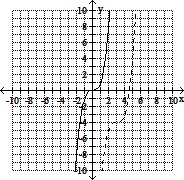
D. 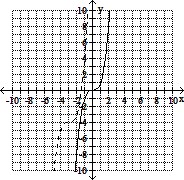
Answer: B
You might also like to view...
An objective function and a system of linear inequalities representing constraints are given. Graph the system of inequalities representing the constraints. Find the value of the objective function at each corner of the graphed region. Use these values to determine the maximum value of the objective function and the values of x and y for which the maximum occurs.Objective Functionz = 9x - 18yConstraints0 ? x ? 5 0 ? y ? 8 4x + 5y ? 30 4x + 3y ? 20
A. Maximum: -78.75; at (1.25, 5) B. Maximum: 0; at (0, 0) C. Maximum: 45; at (5, 0) D. Maximum: -108; at (0, 6)
Solve the problem.A pyramid was constructed for a mini golf center which featured the man-made wonders of the world. The pyramid is depicted in the figure below. Find the length x of a slant edge of the pyramid. Round your answer to the nearest hundredth.
 170.5 inches
170.5 inches
A. 112.69 inches B. 165.03 inches C. 221.44 inches D. 27,233.25 inches
Divide. Write the answer in simplest form.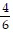 ÷ 7
÷ 7
A. 4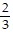
B. 1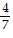
C. 
D. 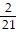
Solve the system mentally, without the use of a calculator or pencil-and-paper calculation. Try to visualize the graphs of both lines.x + 0y = 90x + y = 4
A. x = 9; y = 4
B. x = 4; y = 9
C. x = 9; y = 2
D. x = 2; y = 