Use mathematical induction to prove the following.1 ? 3 + 2 ? 4 + 3 ? 5 + . . . + n(n + 2) =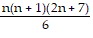 
What will be an ideal response?
Answers may vary. One possibility:
Sn: 1 ? 3 + 2 ? 4 + 3 ? 5 + . . . + n(n + 2) =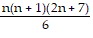 
S1: 1 ? 3 =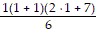 
Sk: 1 ? 3 + 2 ? 4 + 3 ? 5 + . . . + k(k + 2) =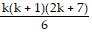 
Sk+1: 1 ? 3 + 2 ? 4 + 3 ? 5 + . . . + k(k + 2) + (k + 1)(k + 3) = 
1. Basis step: Since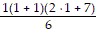 
= 
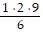= 1 ? 3, S1 is true.
2. Induction step: Let k be any natural number. Assume Sk. Deduce Sk+1.
1 ? 3 + 2 ? 4 + 3 ? 5 + . . . + k(k + 2) =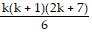 
1 ? 3 + 2 ? 4 + 3 ? 5 + . . . + k(k + 2) + (k + 1)(k + 3) =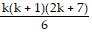 
+ (k + 1)(k + 3)
= 
+ 
= 
+ 
= 
= 
= 
= 
= 
.
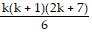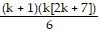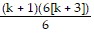
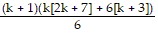
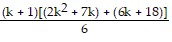
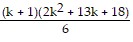
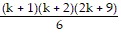
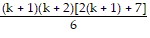You might also like to view...
Find all local extreme values of the given function and identify each as a local maximum, local minimum, or saddle point.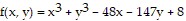
A. (-4, -7); local maximum; (4, 7) local minimum B. (4, -7); saddle point; (-4, 7); saddle point C. (4, 7); local minimum; (4, -7); saddle point; (-4, 7); saddle point; (-4, -7); local maximum D. (-4, -7); local maximum
Provide an appropriate response.Express in scientific notation: 0.000004
A. 4 × 10-5 B. 0.4 × 10-5 C. 4 × 10-6 D. 40 × 10-7
Graph the function.y = -2 cot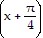 
A.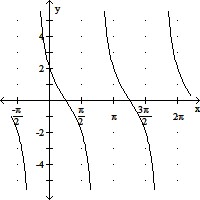 
B. 
C.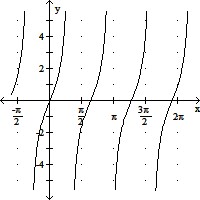 
D. 
Perform the indicated operation and simplify. Write the answer in the form a + bi.(5 - 8i)2
A. -39 B. 10 - 16i C. -39 - 80i D. 25 + 64i