Use the x-intercepts to find the intervals on which the graph of f is above and below the x-axis.f(x) = (x + 15)2
A. above the x-axis: (-?, -15), (-15, ?)
below the x-axis: no intervals
B. above the x-axis: (-15, ?)
below the x-axis: (-?, -15)
C. above the x-axis: (-?, -15)
below the x-axis: (-15, ?)
D. above the x-axis: no intervals
below the x-axis: (-?, -15), (-15, ?)
Answer: A
You might also like to view...
Combine like terms in the expression. Answer "not possible" if terms cannot be combined.78x - y
A. 15xy B. not possible C. 30x - y D. 56y
Find all the roots of the function. Use preliminary analysis and graphing to determine good initial approximations. Round to six decimal places.f(x) = ex +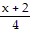 
A. x ? -2.372856 B. x ? -2.872856 C. x ? -2.372745 D. x ? -1.372856
The position vector of a particle is r(t). Find the requested vector.The acceleration at t = 
for r(t) = (10 sin 5t)i - (7 cos 5t)j + (10 csc 5t)k
A. a
= 175j + 250k
B. a
= -250i - 250k
C. a
= -250i + 250k
D. a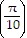
= 250i + 250k
Multiply and simplify.sin ? (sec ? tan ? + csc ? + cot ?)
A. 1 + 2 sin2? B. sin2? - 1 + cos ? C. sin ? + 2 sec ? D. sec2? + cos ?