Solve the problem.A stone is thrown into a pond. A circular ripple is spreading over the pond in such a way that the radius is increasing at the rate of 4.1 feet per second, modeled by the function r(t) = 4.1t. The area of a circular ripple is given by the function A(x) = ?x2, where x is the radius of the circle. Express the area of the ripple as a (composite) function of the number of seconds that have passed since the stone entered the pond.
A. A(r(t)) = 4.1?t2
B. A(r(t)) = 8.2?t2
C. A(r(t)) = 16.81?2t
D. A(r(t)) = 16.81?t2
Answer: D
You might also like to view...
Evaluate the improper integral.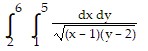
A. 12 B. 24 C. 16 D. 20
Find the standard deviation of the data.Christine is currently taking college astronomy. The instructor often gives quizzes. On the past seven quizzes, Christine got the following scores. Find the standard deviation. Round to the nearest tenth.47 11 39 21 15 44 63
A. 8228.6 B. 39 C. 10,422 D. 19.1
Solve the problem.Two points A and B are on opposite sides of a building. A surveyor selects a third point C to place a transit. Point C is 50 feet from point A and 74 feet from point B. The angle ACB is 53°. How far apart are points A and B?
A. 101 ft B. 75.8 ft C. 111.5 ft D. 59.4 ft
Find the requested term in the sequence.The 10th term in 13, 19, 20, 21, 22, . . .
A. 28 B. 27 C. 26 D. 29