Solve.A plane flying with a tailwind flew at a speed of 420 mph, relative to the ground. When flying against the tailwind, it flew at a speed of 300 mph. Express these relationships as equations. Let x represent the speed of the plane in calm air and let y represent the speed of the wind.
A. x + y = 420
y = 300
B. x + y = 300
x - y = 420
C. x + y = 300
y = 420
D. x + y = 420
x - y = 300
Answer: D
Mathematics
You might also like to view...
Multiply or divide (as specified) the numerator and denominator by the given factor to obtain an equivalent fraction. (divide by x + 1)
(divide by x + 1)
A. 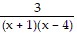
B. 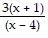
C. 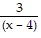
D. 
Mathematics
Use reference angles to find the exact value of the expression. Do not use a calculator.cos 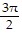
A. 1 B. 0 C. -1 D. undefined
Mathematics
Find the inverse of the one-to-one function.f(x) =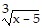
A. f-1(x) = 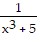
B. f-1(x) = x3 + 5
C. f-1(x) = x + 5
D. f-1(x) = x3 + 25
Mathematics
Evaluate the function.For f(x) = -3x - 22, find f(-8).
A. 2 B. -22 C. 4 D. -8
Mathematics