Solve the problem.Suppose an isolated island has a native population of 10,000 and a person from a visiting ship introduces a disease which has an infection rate of 0.00005. Assume that the rate of spread of the disease satisfies the following logistic equation: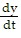 
= k
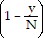y, where N is the size of the population and y is the number infected at time t.How many individuals are infected after 30 days?
A. 9770
B. 9970
C. 9670
D. 9870
Answer: B
You might also like to view...
Solve the problem.Find the point on the curve x = 4 sin t, y = cos 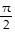t, - 
? t ? 
, closest to the point 
. (Hint: Minimize the square of the distance as a function of t.)
A. 
B. (-4, 0)
C. 
D. (4, 0)
The point P(x, y) on the unit circle that corresponds to a real number t is given. Find the values of the indicated trigonometric function at t.
?Find csc t.
A.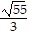 
B. - 
C. - 
D. 
Solve the equation.4z + 17 = 3z + 3
A. z = -14 B. z = -20 C. z = 20 D. z = 14
Solve the problem.A motorcycle and a car leave an intersection at the same time. The motorcycle heads north at an average speed of 20 miles per hour, while the car heads east at an average speed of 48 miles per hour. Find an expression for their distance apart in miles at the end of t hours.
A. t
miles
B. 52
miles
C. 2t
miles
D. 52t miles