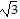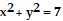Given that f(x) = sin x, g(x) = cos x, and h(x) = tan x, evaluate the given function. The point (x, ), on the circle , also lies on the terminal side of an angle ? in quadrant II. The point , on the circle , also lies on the terminal side of an angle ? in quadrant III.f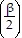

alt="" style="vertical-align: -17.0px;" />



A.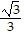  
B. -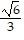 

C. -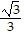 
D.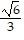  

---

Answer: D

Mathematics

Multiply the monomial and the polynomial.6x3(-7x6 - 3x2 + 12)

A. -42x6 - 18x2 + 72 B. -42x9 - 18x5 C. -42x9 - 3x2 + 12 D. -42x9 - 18x5 + 72x3

Find the indicated composite for the pair of functions.f(x) = 3x + 9; g(x) = 3x - 1Find (f?g)(x).

A. 9x + 8 B. 9x + 12 C. 9x + 6 D. 9x + 26

Solve the problem.The graph of a function is given below. Tell whether the graph could possibly be the graph of a polynomial function. If it could be the graph of a polynomial function, tell which of the following are possible degrees for the polynomial function: 3, 4, 5, 6.

A. yes; 5, 6 B. yes; 3, 5 C. no D. yes; 5 only

For those which are polynomial functions, find the degree, the leading term, and the leading coefficient.f(x) = 3x4 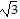+ 5 - x5

A. not a polynomial function
B. Degree: 4, leading term: -x⁵, leading coefficient: -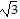
C. Degree: 5, leading term: -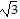x⁵, leading coefficient: -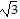
D. Degree: 5, leading term: 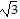x⁵, leading coefficient: