The table below gives the quantity of a product demanded and the quantity supplied for various prices. Solve the problem.Find the linear equation that gives the price as a function of the quantity demanded.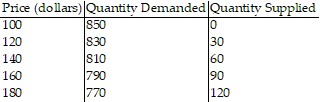
A. p = -q + 950
B. p = 
q + 100
C. p = q + 950
D. p = -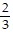 
q + 100
Answer: A
You might also like to view...
Write as an improper fraction.24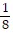
A.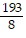 
B. 
C.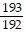 
D.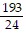 
Solve the inequality. Write the solution in interval notation and graph the solution set.3x + 4 < 25
A. (-?, 7]
B. [7, ?)
C. (-?, 7)
D. (7, ?)
Use slack variables to convert the constraints into linear equations.Maximize3x + 5ysubject to:7x + 9y ? 30 x + 6y ? 40with:x ? 0, y ? 0
A. 7x + 9y + u = 30 x + 6y + u = 40 -3x - 5y + M = 0 Maximize M given that x ? 0, y ? 0, u ? 0 B. 7x + 9y + u = 30 x + 6y + v = 40 -3x - 5y + M = 0 Maximize M given that x ? 0, y ? 0, u ? 0, v ? 0 C. 7x + 9y = u + 30 x + 6y = v + 40 3x + 5y + M = 0 Maximize M given that x ? 0, y ? 0, u ? 0, v ? 0 D. 7x + 9y + u ? 30 x + 6y + v ? 40 3x + 5y + M ? 0 Maximize M given that x ? 0, y ? 0, u ? 0, v ? 0
A polynomial P(x) and a divisor d(x) are given. Use long division to find the quotient Q(x) and the remainder R(x) when P(x) is divided by d(x), and express P(x) in the form d(x)? Q(x) + R(x).P(x) = 3x3 - x2 + 2x + 6d(x) = x + 1
A. (x + 1)(3x2 + 2x) + 6 B. (x + 1)(3x2 - 4x + 2) + 8 C. (x - 1)(3x2 + 2x) + 6 D. (x + 1)(3x2 - 4x + 6) + 0