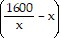The annual spending by tourists in a resort city is 900 million dollars. Approximately 75% of that revenue is again spent in the resort city, and of that amount approximately 75% is again spent in the resort city. If this pattern continues, write the geometric series that gives the total amount of spending generated by the 900 million dollars (including the initial outlay of 900 million dollars) and find the sum of the series.
A. The geometric series is 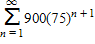
The sum of the series is $3600 million.
B. The geometric series is
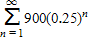 .
.The sum of the series is $67,500 million.
C. The geometric series is
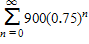 .
.The sum of the series is 3600 million.
D. The geometric series is
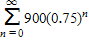 .
.The sum of the series is $67,500 million.
E. The geometric series is
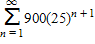 .
.The sum of the series is $675 million.
Answer: C
You might also like to view...
Solve the problem.Estimate the volume of material in a cylindrical shell with height 30 in., radius 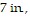 and shell thickness 0.5 in. (Use 3.14 for ?.)
and shell thickness 0.5 in. (Use 3.14 for ?.)
A. 669.4 in.3 B. 329.7 in.3 C. 659.4 in.3 D. 1318.8 in.3
Add or subtract. Express the answer as a mixed number.3 + 17
+ 17
A. 21
B. 19
C. 20
D. 3
Perform the indicated operation.(3a + 10c)(3a - 10c)
A. 3a2 - 10c2 B. 9a2 - 100c2 C. 9a2 + 60ac - 100c2 D. 9a2 - 60ac - 100c2
Solve.Acme Computer Products finds that the marginal revenue R in producing x computer networking connectors is given by the equation 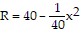 dollars/connector. Find an equivalent expression for R by factoring out
dollars/connector. Find an equivalent expression for R by factoring out 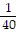 x.
x.
A. R =  x
x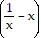
B. R = 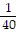 x(1600 - x)
x(1600 - x)
C. R = 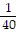 x
x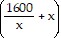
D. R = 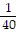 x
x