The smaller the curvature in a bend of a road, the faster a car can travel. Assume that the maximum speed around a turn is inversely proportional to the square root of the curvature. A car moving on the path 
(x and y are measured in miles) can safely go 35 miles per hour at
variablename="impvar_1290da8f700f4fd4a29454f27" />. How fast can it go at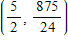
? Round your answer to two decimal places.
?
A. ?86.42 mi/h
B. ?61.11 mi/h
C. ?52.38 mi/h
D. 50.03 mi/h
E. 73.04 mi/h
Answer: A
You might also like to view...
Solve the problem.Find the average height of the paraboloid 
above the annular region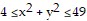 
in the 
.
A.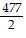 
B.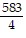 
C. 
D.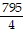 
Find the perimeter (P) of each figure.
A. 24 m B. 33 m C. 26 m D. 25 m
Solve the problem.A parallelogram has a base of length 3x + 1 and a height of x + 1 and has an area of 96 square units. Find the base and height of the parallelogram. (A = bh)
A. height = 4 units; base = 24 units B. height = 6 units; base = 16 units C. height = 2 units; base = 48 units D. height = 8 units; base = 12 units
Assume that the demand function for a certain commodity has the form
?
?
where x is the quantity demanded, measured in units of a thousand, and p is the unit price in dollars. Suppose the quantity demanded is 7,000 (x = 7) when the unit price is $9.00 and 9,000 (x = 9) when the unit price is $7.00. Determine the demand equation.
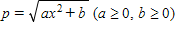
What will be an ideal response?