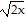Solve the problem.Formulate the following problem as a linear programming problem (DO NOT SOLVE):A veterinarian wants to set up a special diet that will contain at least 500 units of vitamin B1 at least 800 units of vitamin B2 and at least 700 units of vitamin B6. She also wants to limit the diet to at most 300 total grams. There are three feed mixes available, mix P, mix Q, and mix R. A gram of mix P contains 3 units of vitamin B1, 5 units of vitamin B2, and 8 units of vitamin B6. A gram of mix Q contains 9 units of vitamin B1, 8 units of vitamin B2, and 6 units of vitamin B6. A gram of mix R contains 7 units of vitamin B1, 6 units of vitamin B2, and 9 units of vitamin B6. Mix P costs $0.10 per gram, mix Q costs $0.12 per gram, and mix R costs $0.21 per gram. How many grams of each mix
should the veterinarian use to satisfy the requirements of the diet at minimal costs? (Let x1 equal the number of grams of mix P, x2 equal the number of grams of mix Q, and x3 equal the number of grams of mix R that are used in the diet).
A.
| Minimize | C = -0.10x1- 0.12x2 - 0.21x3 |
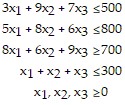
B.
| Minimize | C = 0.10x1- 0.12x2 + 0.21x3 |

C.
| Minimize | C = 0.10x1 + 0.12x2 + 0.21x3 |

D.
| Minimize | C = 0.10x1- 0.12x2 - 0.21x3 |
Answer: C
You might also like to view...
Decide whether the statement is true or false.If the radius of a sphere is doubled, the surface area is multiplied by a factor of 4.
A. True B. False
Decide whether or not the given equation is an identity.sin2 ? - cos2 ? = 2 sin2 ? - 1
A. Yes B. No
Solve the problem.Which apportionment method does not violate the quota rule, and does not suffer from any of the paradoxes?
A. Webster's method B. Jefferson's method C. Hamilton's method D. Adam's method E. There is no such method.
Combine and simplify, if possible.7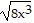 - 7x
- 7x
A. -14x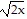
B. -84x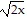
C. -84x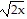 + 7x2
+ 7x2
D. -14x2