Solve the problem.A vineyard produces two special wines, a white and a red. A bottle of the white wine requires 14 pounds of grapes and 1 hour of processing time. A bottle of red wine requires 25 pounds of grapes and 2 hours of processing time. The vineyard has on hand 2,198 pounds of grapes and can allot 160 hours of processing time to the production of these wines. A bottle of the white wine sells for $11.00, while a bottle of the red wine sells for $20.00. How many bottles of each type should the vineyard produce in order to maximize gross sales?
A. 42 bottles of white and 59 bottles of red
B. 76 bottles of white and 42 bottles of red
C. 132 bottles of white and 14 bottles of red
D. 14 bottles of white and 132 bottles of red
Answer: C
You might also like to view...
Solve the problem.Water is falling on a surface, wetting a circular area that is expanding at a rate of 
How fast is the radius of the wetted area expanding when the radius is 
(Round your answer to four decimal places.)
A. 0.0051 mm/s B. 0.0026 mm/s C. 391.6516 mm/s D. 0.0160 mm/s
Solve.Is it possible to find a Maclaurin expansion for f(x) =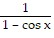 
? Explain.
A. No, the function is not defined at x = 0. B. Yes, the function has derivatives of all orders at x = 0.
Solve the system of equations using matrices.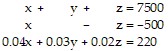
A. (1500, 4000, 2000) B. (1500, 5000, 1000) C. (1000, 4000, 1500) D. (2000, 3500, 2000)
Complete the table.InputOutput16.5 × 1 - 0.8 =26.5 × 2 - 0.8 =36.5 × 3 - 0.8 =46.5 × 4 - 0.8 =
A.
| Input | Output |
| 1 | 6.5 × 1 - 0.8 = 6.7 |
| 2 | 6.5 × 2 - 0.8 = 7.7 |
| 3 | 6.5 × 3 - 0.8 = 8.7 |
| 4 | 6.5 × 4 - 0.8 = 9.7 |
B.
| Input | Output |
| 1 | 6.5 × 1 - 0.8 = 5.7 |
| 2 | 6.5 × 2 - 0.8 = 4.9 |
| 3 | 6.5 × 3 - 0.8 = 4.1 |
| 4 | 6.5 × 4 - 0.8 = 3.3 |
C.
| Input | Output |
| 1 | 6.5 × 1 - 0.8 = 7.3 |
| 2 | 6.5 × 2 - 0.8 = 13.8 |
| 3 | 6.5 × 3 - 0.8 = 20.3 |
| 4 | 6.5 × 4 - 0.8 = 26.8 |
D.
| Input | Output |
| 1 | 6.5 × 1 - 0.8 = 5.7 |
| 2 | 6.5 × 2 - 0.8 = 12.2 |
| 3 | 6.5 × 3 - 0.8 = 18.7 |
| 4 | 6.5 × 4 - 0.8 = 25.2 |