A sausage company makes two different kinds of hot dogs, regular and all beef. Each pound of all-beef hot dogs requires 0.75 lb of beef and 0.2 lb of spices, and each pound of regular hot dogs requires 0.18 lb of beef, 0.3 lb of pork, and 0.2 lb of spices. Suppliers can deliver at most 1,020 lb of beef, at most 600 lb of pork, and at least 500 lb of spices. If the profit is $0.70 on each pound of all-beef hot dogs and $0.40 on each pound of regular hot dogs, how many pounds of each should be produced to obtain maximum profit? What is the maximum profit? Round your profit to the nearest cent, another answers - to the nearest wholenumber.
?
A. Maximum profit of $1150.00 with 2000 lbs of regular and 500 lbs of all beef.
B. Maximum profit of $1416.00 with 2000 lbs of regular and 880 lbs of all beef.
C. Maximum profit of $1752.00 with 880 lbs of regular and 2000 lbs of all beef.
D. Maximum profit of $1300.00 with 1500 lbs of regular and 1000 lbs of all beef.
E. Maximum profit of $1450.00 with 1000 lbs of regular and 1500 lbs of all beef.
Answer: B
You might also like to view...
Simplify the algebraic expression by combining similar terms.3.8x - 7.2y - 6.5x + 14.1y
A. -2.7x + 6.5y B. -2.7x + 7.2y C. -2.8x + 6.9y D. -2.9x + 6.9y E. -2.7x + 6.9y
How many subsets and proper subsets are in the given set?{1, 3, 5, 7, 9, 11}
A. 63, 62 B. 63, 64 C. 64, 63 D. 62, 63
Subtract.(3.1x3 - 6.1x2 + x - 4) - (8.1x3 - 2.7x2 + x + 1)
A. 11.2x3 - 3.4x2 - 5 B. -5x3 - 3.4x2 - 2x + 5 C. -5x3 - 8.8x2 + 2x - 5 D. -5x3 - 3.4x2 - 5
Express the given function H as a composition of two functions f and g such that H(x) = (f ? g)(x).H(x) = 
A. f(x) = 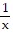 , g(x) = x2 - 8
, g(x) = x2 - 8
B. f(x) = 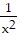 , g(x) = x - 8
, g(x) = x - 8
C. f(x) = 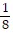 , g(x) = x2 - 8
, g(x) = x2 - 8
D. f(x) = 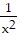 , g(x) = -
, g(x) = - 