Solve the problem.A nuclear scientist has a sample of 
of a radioactive material which has a half-life in hours. She monitors the amount of radioactive material over a period of 30 hours and obtains the following data. Use a graphing utility to fit an exponential function to the data. Round the values to the nearest thousandth. Then, use the function to predict the amount of material, rounded to the nearest tenth, remaining at 40 hours. 
.
A. y = 99.671e0.077x, 2168.7 mg
B. y = 99.671e-0.077x, 6.7 mg
C. y = 99.671e0.926x, 1.2 mg
D. y = 99.671e-0.077x, 4.6 mg
Answer: D
You might also like to view...
Solve the problem.Roberto invested some money at 8%, and then invested $3000 more than twice this amount at 11%. His total annual income from the interest was $3930. How much was invested at 11%?
A. $27,000 B. $9000 C. $24,000 D. $2700
State the name of the property illustrated.(x + 3) + [-(x + 3)] = 0
A. Inverse property of addition B. Commutative property of addition C. Identity property of multiplication D. Inverse property of multiplication
Write the partial fraction decomposition of the rational expression.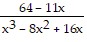
A. 
+ 
+ 
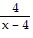B. 
+ 
+ 
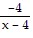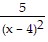
C. 
+ 
+ 
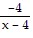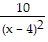
D. 
+ 
+ 
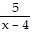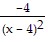
Factor out the GCF from the polynomial.10a(a - b) + (a - b)
A. (a - b)(10a + 1) B. (10a2 - 10ab) + (a - b) C. 10a(a - b) D. 11a(a - b)