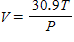The volume V (in liters) of a certain mass of gas is related to its pressure P (in millimeters of mercury) and its temperature T (in degrees Kelvin) by the law
?
?
Compute 
and 
when T = 260 and P = 700.
?
A. 
The volume increases by 0.044 L when the temperature decreases by 1 degree (beyond 260 K) and the pressure is fixed at 700 mm of mercury. The volume decreases by 0.016 L when the pressure increases by 1 mm of mercury (beyond 700 mm) and the temperature is fixed at 260 K.
?
B.
The volume increases by 0.044 L when the temperature increases by 1 degree (beyond 260 K) and the pressure is fixed at 700 mm of mercury. The volume decreases by 0.016 L when the pressure increases by 1 mm of mercury (beyond 700 mm) and the temperature is fixed at 260 K.
?
C.
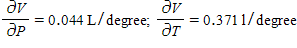
The volume increases by 0.044 L when the temperature increases by 1 degree (beyond 260 K) and the pressure is fixed at 700 mm of mercury. The volume increases by 0.016 L when the pressure increases by 1 mm of mercury (beyond 700 mm) and the temperature is fixed at 260 K.
?
D. ?
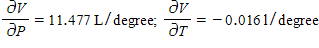
The volume increases by 0.044 L when the temperature increases by 1 degree (beyond 260 K) and the pressure is fixed at 700 mm of mercury. The volume increases by 0.016 L when the pressure increases by 1 mm of mercury (beyond 700 mm) and the temperature is fixed at 260 K.
?
Answer: C
You might also like to view...
Provide an appropriate response. Write the set x > -1 using interval notation.
A. (-?, -1) B. ( -1, ?) C. [ -1, ?) D. (-?, -1]
Express the area of the region bounded by the given line(s) and/or curve(s) as an iterated double integral.The lines 
, 
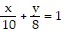, and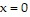 
A.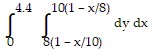 
B.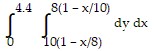 
C.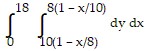 
D. 
The data table has been generated by a linear, quadratic, or cubic function f. All zeros of f are real numbers located in the interval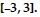 
By making a line graph of the data, conjecture the degree of f.
A. 1 B. 2 C. 3 D. 4
Graph the function by starting with a function from the library of functions and then using the techniques of shifting, compressing, stretching, and/or reflecting.g(x) = (x - 4)2 - 3
A. 
B. 
C. 
D. 