Solve the problem.The grade resistance F of a car traveling up or down a hill is modeled by the equation F = W sin ?, where W is the weight of the car and ? is the angle of the hill's grade (? > 0 for uphill travel, ? < 0 for downhill travel). What is the grade resistance (to the nearest pound) of a 3000-lb car traveling uphill on a 3° grade (
)?
A. 157 lb
B. 3003 lb
C. -3003 lb
D. -157 lb
Answer: A
You might also like to view...
Graph the function f(x) over the given interval. Partition the interval into 4 subintervals of equal length. Then add to your sketch the rectangles associated with the Riemann sum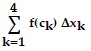 
, using the indicated point in the kth subinterval for ck.f(x) = -2x - 1, [0, 2], left-hand endpoint
A.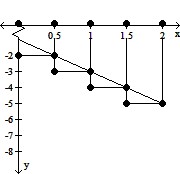 
B.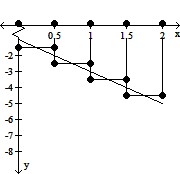 
C.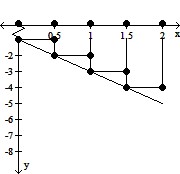 
D.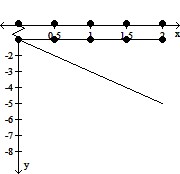 
Find the required part of the geometric sequence.Find a formula for the nth term of a geometric sequence with third term -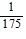 
and sixth term - 
.
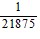A. an =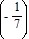 
n - 1 +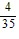 
B. an = -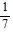 
+ 
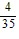(n - 1)
C. an = -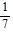 
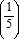n - 1
D. an = -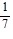 
+ 
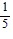(n - 1)
Answer the question.The binomial 64x2 + 256 is the sum of two squares that can be factored. Why is this possible?
What will be an ideal response?
Solve the problem.A system for tracking ships indicated that a ship lies on a hyperbolic path described by 
The process is repeated and the ship is found to lie on a hyperbolic path described by 
If it is known that the ship is located in the first quadrant of the coordinate system, determine its exact location.
A. (-6, -4) B. (4, 6) C. (6, 4) D. (-4, -6)