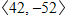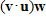Solve the problem.A rocket is shot straight up in the air from the ground at a rate of 68 feet per second. The rocket is tracked by a range finder that is 485 feet from the launch pad. Let d represent the distance from the rocket to the range finder and t represent the time, in seconds, since "blastoff". Express d as a function of t.
A. d(t) = 4852 + (68t)2
B. d(t) = 485 + 68t2
C. d(t) =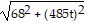 
D. d(t) =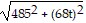 
Answer: D
You might also like to view...
Find the standard deviation s for the given data. Round your answer to one more decimal place than the original data.The manager of a small dry cleaner employs six people. As part of their personnel file, she asked each one to record to the nearest one-tenth of a mile the distance they travel one way from home to work. The six distances are listed below:17.6?15.4?43.4?46.3?18.5?24.7Compute the standard deviation(s).
A. 13.71 B. 4,587.1 C. 5,526.5 D. 44.9
Rotate the axes so that the new equation contains no xy-term. Discuss the new equation.31x2 + 10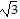
xy + 21y2 -144 = 0
A. ? = 45°
y'2 = -4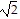
x'
parabola
vertex at (0, 0)
focus at (-
, 0)
B. ? = 45°
x'2 = -4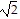
y'
parabola
vertex at (0, 0)
focus at (0, -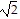
)
C. ? = 30°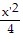
+ 
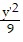= 1
ellipse
center at (0, 0)
major axis is y'-axis
vertices at (0, ±3)
D. ? = 36.9°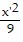
+ 
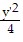= 1
ellipse
center at (0, 0)
major axis is x'-axis
vertices at (±3, 0)
Solve the equation by the square root property. If possible, simplify radicals or rationalize denominators. Express imaginary solutions in the form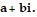 
6x2 = 96
A. {0}
B. {±4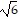
}
C. {±4}
D. {±6}
Use the vectors 
, 
, and 
class="wirisformula" align="middle" style="vertical-align: middle;" data-wiris-created="true" varid="variable_id_field" variablename="impvar_4156526c5f3b4180b7bf9911b" /> to find the indicated quantity. State
whether the result is a vector or a scalar.
?
?
A.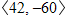 
; vector
B. 
; vector
C. -58; scalar
D. -56; scalar
E. 
; vector