Use the graph of the function f(x) to locate the local extrema and identify the intervals where the function is concave up and concave down.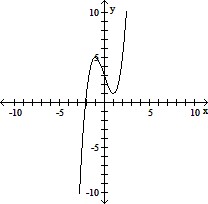
A. Local minimum at x = 1; local maximum at x = -1; concave up on (0, ?); concave down on (-?, 0)
B. Local minimum at x = 1; local maximum at x = -1; concave down on (-?, ?)
C. Local minimum at x = 1; local maximum at x = -1; concave down on (0, ?); concave up on (-?, 0)
D. Local minimum at x = 1; local maximum at x = -1; concave up on (-?, ?)
Answer: A
You might also like to view...
Use Fleury's algorithm to find an Euler circuit for the graph beginning and ending at the indicated vertex. If no Euler circuit exists, state this.Using the following graph, find an Euler circuit that begins and ends with vertex B.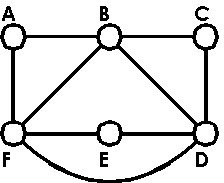
A. B ? C ? D ? E ? F ? A ? B ? C ? D ? B ? F ? D ? B B. No Euler circuit exists. C. B ? C ? D ? E ? F ? A ? B ? D ? F ? B D. B ? A ? F ? E ? D ? C ? B
Solve the equation symbolically.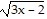 +
+ 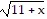 = -1
= -1
A. - 
B. 5
C. No solution
D. 0
Find the value of the determinant.
A. -236 B. 236 C. -92 D. 36
Subtract. Use borrowing, if necessary.
A. 83 B. 85 C. 37 D. 45