Solve the problem.The unit vectors u and v are combined to produce two new vectors 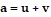 and
and 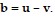 Show that a and b are orthogonal. Assume
Show that a and b are orthogonal. Assume 
What will be an ideal response?
u = ux i + uyj and v = vx i + vyj , so
a = u + v = (ux + vx) i + (uy + vy)j and b = u - v = (ux - vx) i + (uy - vy)j
Take the dot product a ? b:
a ? b = (u + v) ? (u - v) = (ux + vx)(ux - vx) + (uy + vy)(uy - vy)
= 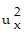 -
-  +
+  -
- 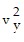 = (
= (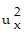 +
+  ) - (
) - (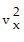 +
+ 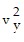 )
)
= 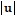 -
- 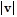 = 1 - 1 = 0
= 1 - 1 = 0
Since the dot product of the two non-zero vectors is zero they are orthogonal.
You might also like to view...
Solve the problem.A land developer wants to develop 8 acres of land. Each lot in the development is to be 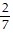 of an acre. How many lots will the land developer have in the 8 acres?
of an acre. How many lots will the land developer have in the 8 acres?
A. 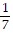 lot
lot
B. 1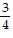 lots
lots
C. 2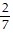 lot(s)
lot(s)
D. 28 lots
Factor. Check by multiplying.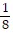 x -
x - 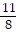 y +
y + 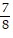
A. 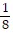 (x - 11y + 7)
(x - 11y + 7)
B. 

C. 8(x - 11y + 7)
D.  (x + 11y - 7)
(x + 11y - 7)
Find the area of the region completely enclosed by the graphs of the given functions f and g.
?

A. ?15 B. ?19 C. ?18 D. ?22 E. ?20
List the intercepts of the graph.Tell whether the graph is symmetric with respect to the x-axis, y-axis, origin, or none of these. 
A. intercepts: (0, -2) and (0, 2) symmetric with respect to x-axis, y-axis, and origin B. intercepts: (-2, 0) and (2, 0) symmetric with respect to origin C. intercepts: (-2, 0) and (2, 0) symmetric with respect to x-axis, y-axis, and origin D. intercepts: (0, -2) and (0, 2) symmetric with respect to y-axis