Determine whether the statement is true or false. If it is true, explain why it is true. If it is false, give an example to show why it is false.
If a1, a2, a3, ... , an and b1, b2, b3, ... , bn are geometric progressions, then a1 b1, a2 b2, a3 b3, ... , an bn is also a geometric progression.
a. It is false.
If
a1, a2, a3, a4, ... = 1, 3, 6, 9,..
b1, b2, b3, b4, ... = 2, 4, 8, 16,..
are geometric progressions, then we can see that
a1 b1, a2 b2, a3 b3, ... = 2, 12, 48, ...
is not a geometric progression.
b. It is true.
A geometric progression is completely determined if the first term and the common ratio are known. Thus, if
a1, a2, a3, ... , an , ...
is a geometric progression with the first term given by a and common ratio given by r, then by definition,
a1 = a
a2 = a1 r = ar
a3 = a2r = ar2
.
.
an = an-1 = ar n – 1
Thus, we see that the product of two nths terms of two geometric progressions is given by
a n b n = a r n – 1 b
where a,b are the first terms of these progressions and r, r1 are their common ratios respectively. Then we
obtain that
a1, b1, a2, b2, a3, b3, ... , an, bn is also geometric progression with first term ab and common ratio rr1.
b. It is true.
A geometric progression is completely determined if the first term and the common ratio are known. Thus, if
a1, a2, a3, ... , an , ...
is a geometric progression with the first term given by a and common ratio given by r, then by definition,
a1 = a
a2 = a1 r = ar
a3 = a2r = ar2
.
.
an = an-1 = ar n – 1
Thus, we see that the product of two nths terms of two geometric progressions is given by
a n b n = a r n – 1 b
where a,b are the first terms of these progressions and r, r1 are their common ratios respectively. Then we
obtain that
a1, b1, a2, b2, a3, b3, ... , an, bn is also geometric progression with first term ab and common ratio rr1.
You might also like to view...
Translate the equation into a sentence by using the phrase "varies directly" or "varies inversely". w = 
A. h varies inversely as the cube of w. B. w varies inversely as the cube root of h. C. w varies directly as the cube of h. D. w varies inversely as the cube of h.
Solve.When a ball with coefficient of restitution e is dropped from a height h meters onto a large flat surface, the time t from the first impact until the ball stops bouncing is given by t = 
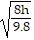 seconds. Find the time it takes a ball with coefficient of restitution 0.21 to stop bouncing if it is dropped from a height of 67.1 centimeters. (Round to the nearest tenth.)
seconds. Find the time it takes a ball with coefficient of restitution 0.21 to stop bouncing if it is dropped from a height of 67.1 centimeters. (Round to the nearest tenth.)
A. 1.9 seconds B. 18.7 seconds C. 27.8 seconds D. 2.8 seconds
Write the fraction as a decimal. If necessary, round to the nearest thousandth.- 
A. -0.52 B. -0.66 C. -0.4 D. -0.6
Solve.Given two 15-cm sides and two 10-cm sides, how many quadrilaterals can be formed with a 75° angle?
What will be an ideal response?