Solve the problem.Find a matrix A and a column matrix B that describe the following tables involving credits and tuition costs. Find the matrix product AB and interpret the significance of the entries of this product.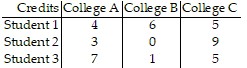
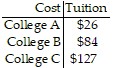
A.
AB = 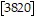
The total tuition paid by all 3 students is $3820.
B.
AB = 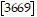
The total tuition paid by all 3 students is $3669.
C.
AB = 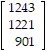
Tuition for Student 1 is $1243, tuition for Student 2 is $1221, and tuition for Student 3 is $901.
D.
AB = 
Tuition for Student 1 is $1236, tuition for Student 2 is $1223, and tuition for Student 3 is $887.
Answer: C
You might also like to view...
Solve the problem.The fuel for a gas snowblower consists of 1.9 parts oil to 39.9 parts gas. Determine the ratio of oil to gas in simplest form.
A. 1 to 22 B. 20 to 1 C. 1 to 21 D. 21 to 1
Solve the problem.Determine whether the ordered triple (7, 2, -5) is a solution of the system of equations.3x - 8y + z = 02x + 4y - 3z = 37-x + 2y - z = 2
A. Yes B. No
Simplify. Write the result with positive exponents only.(5p3q6)-2(25p5q3)2
A. 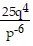
B. 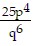
C. 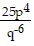
D. 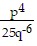
Solve the problem.A lumber yard has fixed costs of $5245.20 per day and variable costs of $0.08 per board-foot produced. Lumber sells for $1.88 per board-foot. How many board-feet must be produced and sold daily to break even?
A. 65,565 board-feet B. 2676 board-feet C. 1942 board-feet D. 2914 board-feet