Use calculus to find any critical points and inflection points of the given function. Then determine the concavity of the function and the intervals over which it is increasing/decreasing.f(x) = e7x
A. Critical points: none
Inflection points: point of inflection at x = 0
Concavity: concave down for all x < 0 and concave up for all x > 0
Increasing: increasing for all real numbers
B. Critical points: critical point at x = 0
Inflection points: none
Concavity: concave up for all real numbers
Increasing: increasing for all x < 0 and decreasing for all x > 0
C. Critical points: none
Inflection points: none
Concavity: concave down for all real numbers
Decreasing: decreasing for all real numbers
D. Critical points: none
Inflection points: none
Concavity: concave up for all real numbers
Increasing: increasing for all real numbers
Answer: D
You might also like to view...
Evaluate the integral.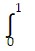
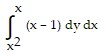
A. 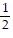
B. - 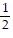
C. - 
D. - 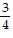
Use the quadratic formula to solve the equation.12y2 = 13y - 3
A. y = 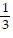 ,
, 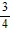
B. y = 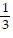 ,
, 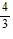
C. y = -  ,
, 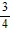
D. y = 3, 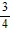
For the compound inequality, give the solution set in both interval and graph forms.5x - 1 < 4 and x - 2 > -1![]()
A. (0, 1)![]()
B. [0, 1]![]()
C. {1}![]()
D. ?![]()
Multiply.-3(-3)(-3)
A. -17 B. -27 C. 27 D. 18