Which of the following is NOT characteristic of a student who functions at the first van Hiele level?
a. Being able to name a square when it is shown to him or her
b. Carefully comparing sides and angles of shapes to one another
c. Believing that squares and rectangles are completely different shapes
d. Thinking that changing the orientation of a square makes it a different shape
Ans: b
You might also like to view...
Find a polar equation for the circle.(x + 10)2 + y2 = 100
A. r cos ? = -20 B. r = -20 sin ? C. r = 20 cos ? D. r = -20 cos ?
Describe the transformations that produce the graph of g from the graph of f.f(x) = x2 ; g(x) = -(x - 9)2 + 2
A. Shift it 9 units to the left. Reflect it across the 
Shift it 2 units up.
B. Shift it 9 units to the right. Reflect it across the 
Shift it 2 units up.
C. Shift it 9 units to the right. Reflect it across the 
Shift it 2 units down.
D. Shift it 9 units to the right. Reflect it across the 
Shift it 2 units up.
Solve the problem.Suppose that the speed of a car, measured in miles per hour (mph), is monitored for some short period of time after the driver applies the brakes. The following table and graph relate the speed of the car to the amount of time, measured in seconds (sec), elapsed from the moment that the brakes are applied. What is happening to the speed of the car during this time frame? In which of the time intervals does the speed change the most?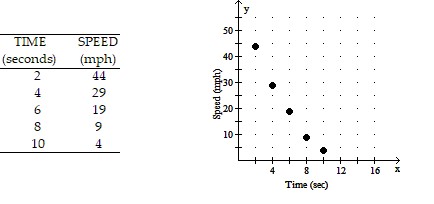
A. With increasing elapsed time, the speed increases. The speed changes most during the time interval from 8 seconds to 10 seconds. B. With increasing elapsed time, the speed decreases. The speed changes most during the time interval from 2 seconds to 4 seconds. C. With increasing elapsed time, the speed decreases. The speed changes most during the time interval from 8 seconds to 10 seconds. D. With increasing elapsed time, the speed increases. The speed changes most during the time interval from 2 seconds to 4 seconds.
Write the word statement as an equation. Use x to represent the unknown number. Do not solve.Four times the sum of a number and 31 decreased by 9 is the same as the difference of six times the number and 15.
A. (4x + 31) - 9 = 6x - 15 B. (4x + 31) - 9 = 15 - 6x C. 4(x + 31) - 9 = 6(x - 15) D. 4(x + 31) - 9 = 6x - 15