Factor the sum or difference of two cubes.729y3z - z
A. z(9y - 1)(81y2 + 1)
B. z(9y + 1)(81y2 - 9y + 1)
C. z(729y - 1)(y2 + 9y + 1)
D. z(9y - 1)(81y2 + 9y + 1)
Answer: D
You might also like to view...
Find the center and radius of the sphere.x2 + (y + 7)2 + (z - 1)2 = 64
A. C(0, 7, -1), a = 64 B. C(0, -7, 1), a = 64 C. C(0, 7, -1), a = 8 D. C(0, -7, 1), a = 8
Solve the problem.In how many ways can you exactly cover the last two diagrams with "dominoes" that are just the size of two small squares?
Use inductive reasoning to investigate the number of ways that a 2 × n strip can be exactly covered with "dominoes".[Hint: consider the Fibonacci numbers F1 = 1, F2 = 1, F3 = 2, F4 = 3, F5 = 5, F6 = 8, F7 = 13, . . . ]
A. 10 ways; 28 ways
The number of ways that a 2 × n strip can be exactly covered with "dominoes" is given by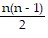 
B. 8 ways; 34 ways
The number of ways that a 2 × n strip can be exactly covered with "dominoes" is given by the Fibonacci number Fn
C. 8 ways; 34 ways
The number of ways that a 2 × n strip can be exactly covered with "dominoes" is given by the Fibonacci number Fn+1
D. 9 ways; 15 ways
The number of ways that a 2 × n strip can be exactly covered with "dominoes" is given by 2n - 1
Find the extreme values of the function subject to the given constraint.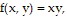
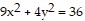
A. Maximum: 3 at 
and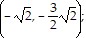 
minimum: -3 at 
and 
B. Maximum: 3 at 
minimum: -3 at 
C. Maximum: 3 at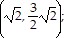 
minimum: -3 at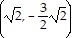 
D. Maximum: 3 at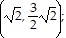 
minimum: -3 at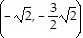 
Find the least common multiple.x2- 4x- 12, 3x- 18
A. 3(x + 2)(x - 6) B. 3(x - 2)(x + 6) C. 3(x - 2)(x - 6) D. 3(x + 2)(x + 6)