Use the Principle of Mathematical Induction to show that the statement is true for all natural numbers n.2 + 2 ?  + 2 ?
+ 2 ?  2 + ... + 2 ?
2 + ... + 2 ?  n - 1 =
n - 1 = 
What will be an ideal response?
First we show that the statement is true when n = 1.
For n = 1, we get 3 = 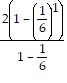 = 3.
= 3.
This is a true statement and Condition I is satisfied.
Next, we assume the statement holds for some k. That is,
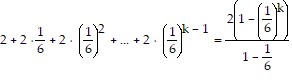 is true for some positive integer k.
is true for some positive integer k.
We need to show that the statement holds for k + 1. That is, we need to show that
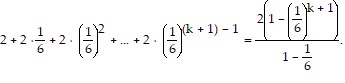
So we assume that 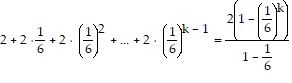 is true and add the next term,
is true and add the next term, 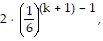 to both sides of the equation.
to both sides of the equation.
2 + 2 ? 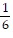 + 2 ?
+ 2 ? 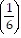 2 + ... + 2 ?
2 + ... + 2 ? 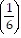 k - 1 + 2 ?
k - 1 + 2 ? 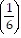 (k + 1) - 1=
(k + 1) - 1= 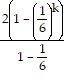 + 2 ?
+ 2 ? 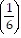 (k + 1) - 1
(k + 1) - 1
= 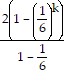 + 2
+ 2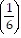 k
k
= 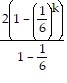 +
+ 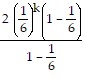
= 
= 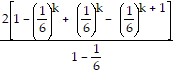
= 
Condition II is satisfied. As a result, the statement is true for all natural numbers n.
You might also like to view...
Use the even-odd identities to find the exact value of the expression. cos (-30°)
A. - 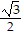
B. - 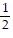
C. 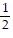
D. 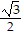
For what values of x does the series converge conditionally?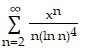
A. x = 1 B. x = -1 C. x = ±1 D. None
Solve the problem.David's company has to ship 3850 boxes of sprinklers. 7 truckloads all containing the same number of boxes were delivered. How many boxes were shipped in each truck?
A. 555 boxes B. 50 boxes C. 55 boxes D. 550 boxes
Match the function f with its graph.f(x) = ln (x)
A. 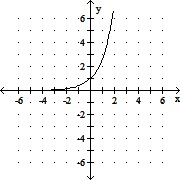
B. 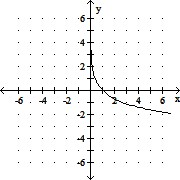
C. 
D. 