A candy maker mixes chocolate, milk, and mint extract to produce three kinds of candy (I, II, and III) with the following proportions. If 75 lb of chocolate, 52 gal of milk, and 36 oz of mint extract are available, how much of each kind of candy can be produced?
?
I : 7 lb chocolate, 5 gal milk, 1 oz mint extract
II : 3 lb chocolate, 2 gal milk, 2 oz mint extract
III : 4 lb chocolate, 3 gal milk, 3 oz mint extract
?
A. 5 units of candy |, 15 units of candy ||, 2 units of candy |||
B. 5 units of candy |, 14 units of candy ||, 2 units of candy |||
C. 4 units of candy |, 16 units of candy ||, 3 units of candy |||
D. 4 units of candy |, 13 units of candy ||, 2 units of candy |||
E. 5 units of candy |, 13 units of candy ||, 3 units of candy |||
Answer: D
You might also like to view...
Use a reciprocal identity to find the indicated trigonometric function of ?.sec ? if cos ? = - 
A. - 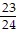
B. 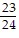
C. 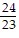
D. - 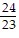
Solve.Five divided by the difference of a number and 9 equals the quotient of 10 and the sum of the number and 3. Find the number.
A. 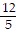
B. -15
C. 7
D. 21
Solve.The cost in millions of dollars for a company to manufacture x thousand automobiles is given by the function C(x) = 3x2 - 18x + 72. Find the number of automobiles that must be produced to minimize the cost.
A. 6 thousand automobiles B. 3 thousand automobiles C. 9 thousand automobiles D. 45 thousand automobiles
Find the values for each of the dimensions of a closed-top box of length x, width y, and height z (in inches) if the volume equals 32,768 cubic inches and the box requires the least amount of material to make. ?
A. x = 46 inches, y = 46 inches, z = 46 inches B. x = 32 inches, y = 32 inches, z = 32 inches C. x = 41 inches, y = 41 inches, z = 41 inches D. x = 45 inches, y = 45 inches, z = 45 inches E. x = 34 inches, y = 34 inches, z = 34 inches