Find an equation of the parabola described.Focus at (0, 15); directrix the line y = -15
A. y2 = 60x
B. x2 = 60y
C. y2 = 15x
D. x2 = -60y
Answer: B
You might also like to view...
Find the partial derivative of the dependent variable or function with respect to each of the independent variables.f(x, y) = x3 - 5x2y - 4xy3
A. 
= 3x2 + 2xy - 4y3; 
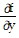= -5x2 + 3xy2
B. 
= 3x2;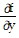 
= -5x2 - 12xy2
C. 
= 3x2 - 10xy - 4y3; 
= -5x2 - 12xy2
D.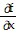 
= x2 - 5xy - 4y3; 
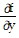= -5x2 - 4xy2
Factor out the requested common factor.Factor out 2x from 8x8/5 - 6x8/5
A. 2x(47/5 - 37/5) B. 2x(48/5 - 38/5) C. 2x(4x7/5 - 3x7/5 ) D. 2x(4x3/5 - 3x3/5)
Use the product rule to simplify. Write the results using exponents.x12 ? x4
A. 2x48 B. x48 C. x16 D. 2x16
Find the vertex, focus, and directrix of the parabola. Graph the equation.x2 = -16y
A. vertex: (0, 0)
focus: (4, 0)
directrix: x = -4
B. vertex: (0, 0)
focus: (0, -4)
directrix: y = 4
C. vertex: (0, 0)
focus: (-4, 0)
directrix: x = 4
D. vertex: (0, 0)
focus: (0, 4)
directrix: y = -4