Find the critical point(s) of the function. Then use the second derivative test to classify the nature of each point, if possible. Finally, determine the relative extrema of the function.
?

?
A. 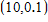

B. 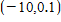
C. 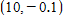
D. 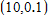

E. no critical points
Answer: C
Mathematics
You might also like to view...
Find the requested part of the triangle. Round your answer to two decimal places.Find the measure of the angle A in degrees. 
A. 34.68° B. 56.62° C. 55.32° D. 54.92°
Mathematics
Determine whether the relation is a function.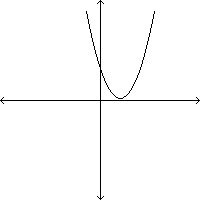
A. Not a function B. Function
Mathematics
Subtract.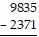
A. 7402 B. 9464 C. 7464 D. 7462
Mathematics
Add.5.8 + (-8.4)
A. 2.6 B. -14.2 C. -2.6 D. 14.2
Mathematics