Find a matrix A and a column matrix B that describe the following tables involving credits and tuition costs. Find the matrix product AB, and interpret the significance of the entries of this product.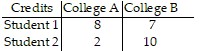
?
A.
AB =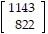 
Tuition for Student 2 is $1143 and tuition for Student 1 is $822.
B.
AB =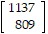 
Tuition for Student 2 is $1137 and tuition for Student 1 is $809.
C.
AB =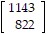 
Tuition for Student 1 is $1143 and tuition for Student 2 is $822.
D.
AB =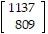 
Tuition for Student 1 is $1137 and tuition for Student 2 is $809.
Answer: C
You might also like to view...
Write the equation of the line in slope-intercept form.m = 
, y-intercept (0, -3)
A. y = 
x + 3
B. y = - 
x - 3
C. y = - 
x + 3
D. y = 
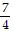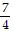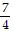x - 3
The given equation cannot be solved symbolically. Find any solutions graphically to the nearest hundredth.log(x - 3) + 3 = 10x
A. -2.99, 0.27 B. 0.27 C. 0.55 D. -2.99, 0.55
For the graph of the function y = f(x), find the absolute maximum and the absolute minimum, if it exists.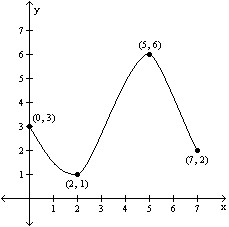
A. Absolute maximum: f(7) = 2; Absolute minimum: f(0) = 3 B. Absolute maximum: f(2) = 7; Absolute minimum: f(3) = 0 C. Absolute maximum: f(5) = 6; Absolute minimum: f(2) = 1 D. Absolute maximum: f(6) = 5; Absolute minimum: f(1) = 2
Multiply.(7x2 + y2 - 7xy)(x2 + 7y2 - xy)
A. 7x4 - 57x2y2 + 14x3y - 7y4 + xy3 - 49x2y3
B. 7x4 + 57x2y2 - 14x3y + 7y4 - 50xy3
C. 7x4 - 57x2y2 + 14x3y - x3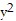
- 7y4 - 50xy3
D. 7x4 + 57x2y2 - 14x3y + x3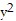
+ 7y4 + xy3 - 49x2y3