Use the accompanying graph of a particle moving on a coordinate line with velocity v = f(t) in ft/sec at time t seconds. The axes are marked off at one-unit intervals. Use these terms to describe the motion state: moving forward/backward, increasing/decreasing speed, and resting. Recall that speed =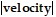 
. 
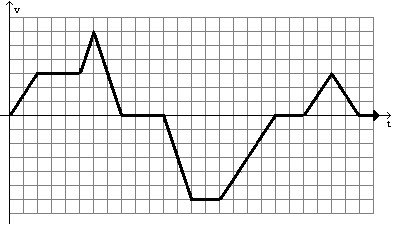Give the interval(s) when the particle is moving forward.
A. (0, 8) and (21, 25)
B. (11, 19)
C. (8, 11) and (19, 21)
D. (0, 11) and (19, 25)
Answer: A
You might also like to view...
Solve the problem.Assume the cost of a car is $21,000. With continuous compounding in effect, find the number of years it would take to double the cost of the car at an annual inflation rate of 6.2%. Round the answer to the nearest hundredth.
A. 160.52 yr B. 1.61 yr C. 11.18 yr D. 171.70 yr
Find the point-slope form of the equation of the line satisfying the given conditions and use this to write the slope-intercept form of the equation.Passing through (3, -7), (-7, 3)
A. y = x - 4 B. y = x + 4 C. y = -x - 4 D. y = -x + 4
Solve the radical equation.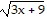
+ 8 = 17
A. ?
B. {216}
C. 
D.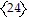 
Solve the problem.To save for retirement, you decide to deposit $1250 into an IRA at the end of each year for the next 40 years. If the interest rate is 9% per year compounded annually, find the value of the IRA after 40 years. (Round to the nearest dollar.)
A. $422,353 B. $15,206,699 C. $386,333 D. $38,012