Find the center, transverse axis, vertices, and foci of the hyperbola.25x2 - 4y2 = 100
A. center at (0, 0)
transverse axis is x-axis
vertices: (0, -2), (0, 2)
foci: (0, -
), (0, 
)
B. center at (0, 0)
transverse axis is x-axis
vertices: (-2, 0), (2, 0)
foci: (-
, 0), (
, 0)
C. center at (0, 0)
transverse axis is x-axis
vertices: (-5, 0), (5, 0)
foci: (-
, 0), (
, 0)
D. center at (0, 0)
transverse axis is x-axis
vertices: (-2, 0), (2, 0)
foci: (-
, 0), (
, 0)
Answer: D
You might also like to view...
Use your grapher to find the surface's area numerically.y = sinx, 0 ? x ? ?/6; x-axis
A. 1.15 B. 5.1 C. 13.39 D. 7.65
Referring to the circuit below, the low pass filter has the following characteristic(s):
a. The output voltage is equal to Vin at fCO
b. The power output is 50% of max. at fCO
c. The output decreases as frequency increases
d. The power output is 50% of max. at fCO and the output decreases as frequency increases
Find the x- and y-intercepts. If no x-intercepts exist, state so.f(x) = x2 - 3
A. x-intercepts (0, 
) and (0, -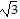
); y-intercept (0,-3)
B. x-intercepts (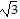
, 0) and (-
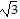, 0); y-intercept (0,3)
C. x-intercepts (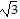
, 0) and (-
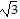, 0); y-intercept (0,-3)
D. x-intercepts (0,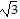 
) and (0, -
); y-intercept (0,3)
Find the inverse of the function.f(x) = 
- 4 for x ? 0
A. f-1(x) = x + 4 B. f-1(x) = -(x + 4)2 C. f-1(x) = (x + 4)2 D. f-1(x) = (x - 4)2