Find the center, transverse axis, vertices, foci, and asymptotes of the hyperbola. -
-  = 1
= 1
A. center at (-4, -3)
transverse axis is parallel to x-axis
vertices at (-10, -3) and (2, -3)
foci at (-4 - 2 , -3) and (-4 + 2
, -3) and (-4 + 2 , -3)
, -3)
asymptotes of y + 3 = -  (x + 4) and y + 3 =
(x + 4) and y + 3 =  (x + 4)
(x + 4)
B. center at (-4, -3)
transverse axis is parallel to y-axis
vertices at (-4, -7) and (-4, 1)
foci at (-4, -3 - 2 ) and (-4, -3 + 2
) and (-4, -3 + 2 )
)
asymptotes of y - 3 = - 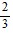 (x - 4) and y - 3 =
(x - 4) and y - 3 =  (x - 4)
(x - 4)
C. center at (-4, -3)
transverse axis is parallel to x-axis
vertices at (-8, -3) and (0, -3)
foci at (-4 - 2 , -3) and (-4 + 2
, -3) and (-4 + 2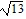 , -3)
, -3)
asymptotes of y + 3 = -  (x + 4) and y + 3 =
(x + 4) and y + 3 = 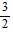 (x + 4)
(x + 4)
D. center at (-3, -4)
transverse axis is parallel to x-axis
vertices at (-7, -4) and (1, -4)
foci at (-3 - 2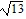 , -4) and (-3 + 2
, -4) and (-3 + 2 , -4)
, -4)
asymptotes of y + 4 = -  (x + 3) and y + 4 =
(x + 3) and y + 4 =  (x + 3)
(x + 3)
Answer: C
You might also like to view...
Evaluate the integral.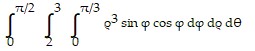
A.  ?
?
B. 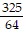 ?
?
C. 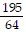 ?
?
D. 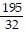 ?
?
Divide. Simplify, if possible.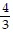 ÷
÷ 
A. 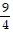
B. 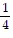
C. 4
D. 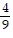
A delivery truck must deliver packages to 6 different store locations (A, B, C, D, E, and F). The trip must start and end at A. The graph below shows the distances (in miles) between locations. We want to minimize the total distance traveled.
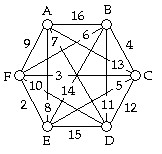 The cheapest-link tour starting with vertex A is given by:
The cheapest-link tour starting with vertex A is given by:
A. A, E, F, C, B, D, A. B. A, C, D, B, E, F, A. C. A, D, F, E, C, B, A. D. A, B, C, D, E, F, A. E. none of these
Rationalize the denominator and simplify.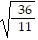
A. 6
B. 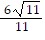
C. 127
D. 