Give an appropriate answer.Show that the function f(x) = x3 + 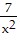 + 2 has exactly one zero on the interval (-?, 0).
+ 2 has exactly one zero on the interval (-?, 0).
What will be an ideal response?
The function f(x) is continuous on the open interval (-?, 0). Also, f(x) approaches -? as x approaches -?, and f(x) approaches ? as x approaches 0 from the left. Since f(x) is continuous and changes sign along the interval, it must have at least one root on the interval.
The first derivative of f(x) is f'(x) = 3x2 - 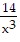 , which is everywhere positive on (-?, 0). Thus, f(x) has a single root on (-?, 0).
, which is everywhere positive on (-?, 0). Thus, f(x) has a single root on (-?, 0).
You might also like to view...
Subtract. Check by adding.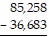
A. 54,575 B. 48,575 C. 48,569 D. 48,469
Use the horizontal line test to determine if the function is one-to-one.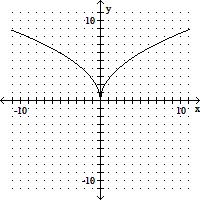
A. No B. Yes
Consider the functions F and G as shown in the graph. Provide an appropriate response.Graph G - F.


A. 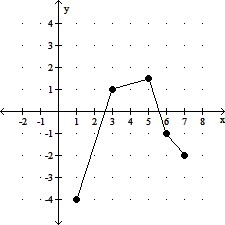
B. 
C. 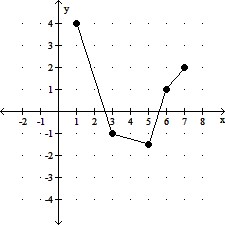
D. 
Use the figure below to solve the right triangle if b= 9 and m?B = 16°. Round all answers to one decimal place.
 FIGURE.png)