Prove that the equation is an identity.cos2(A - B) - cos2(A + B) = sin 2A sin 2B
What will be an ideal response?
cos2(A - B) - cos2(A + B) = (cos A cos B + sin A sin B)2 - (cos A cosB - sin A sin B)2
= (cos2A cos2B + 2 cos A cos B sin A sin B + sin2A sin2B) -
(cos2A cos2B - 2 cos A cos B sin A sin B + sin2A sin2B)
= 4 cos A cos B sin A sin B
= (2 sin A cos A) ? (2 sin B cos B)
= sin 2A sin 2B Using the double-angle identity
You might also like to view...
Solve the problem.The following map shows the states Illinois, Indiana, Kentucky, West Virginia, and Virginia. Find a route that starts and ends in Virginia and crosses each common state border exactly one time.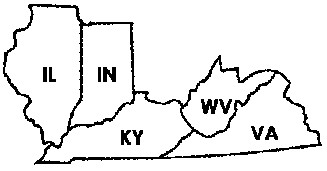
A. VA ? KY ? IL ? IN ? KY ? VA ? WV ? KY B. VA ? WV ? KY ? IL ? IN ? KY C. No such route exists. D. VA ? WV ? KY ? IN ? IL ? KY ? VA
Provide an appropriate response.The payoff table for three possible courses of action A1, A2, and A3 is given below.pi?A1xi?A2xi?A3xi?.3$70$40$50.2$100$120$110.1$160$140$90.4$80$140$160Which course of action will produce the largest expected value? What is it?
What will be an ideal response?
Simplify the expression. Assume that all variables are positive when they appear. -
- 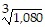 +
+ 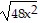
A. 5x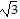 - 6
- 6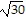
B. 5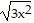 -
- 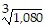
C. 5x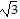 - 6
- 6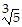
D. 4x - 6
- 6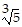
Add.8n5 - 2n - 7n2 and 9n2 + 4n5 - 8n
A. 12n + 2n5 - 10n2 B. 2n5 + 17n2 - 15n C. 12n5 + 2n2 - 10n D. 4n8