Use the Principle of Mathematical Induction to show that the statement is true for all natural numbers n.1 + 5 + 52 + ... + 5n - 1 = 
What will be an ideal response?
First, we show that the statement is true when n = 1.
For n = 1, we get 1 (or 5[(1) - 1]) = 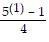 =
= 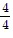 = 1.
= 1.
This is a true statement and Condition I is satisfied.
Next, we assume the statement holds for some k. That is,
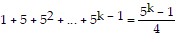 is true for some positive integer k.
is true for some positive integer k.
We need to show that the statement holds for 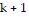 . That is, we need to show that
. That is, we need to show that
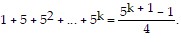
So we assume that 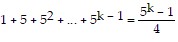 is true and add the next term, 5k, to both sides of the equation.
is true and add the next term, 5k, to both sides of the equation.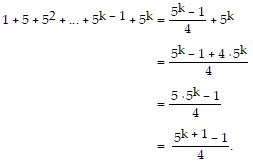
Condition II is satisfied. As a result, the statement is true for all natural numbers n.
You might also like to view...
The wages of the employees of a company are presented in this histogram. Answer the question. How many employees earn at least $4 and less than $6 an hour?
How many employees earn at least $4 and less than $6 an hour?
A. 10 B. 20 C. 34 D. 22
Perform the indicated operations and simplify the result. Leave the answer in factored form. ?
? 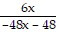
A. - 
B. - 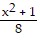
C. - 
D. 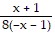
Provide an appropriate response.Explain how the graph of f(x) = ln x could be used to graph the function g(x) = ex - 1.
What will be an ideal response?
Graph y = f(x).f(x) =  x - 3
x - 3
A. 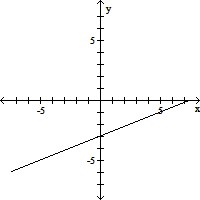
B. 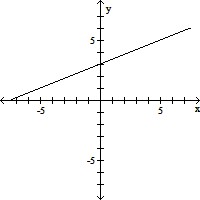
C. 
D. 