Use the graph of the function to estimate the x-intercepts.g(x) = -8x2 + 18x + 5
A. x = 0.25, x = 2.5
B. x = -2.5, x = 0.25
C. x = 5, x = 18
D. x = -0.25, x = 2.5
Answer: D
You might also like to view...
Solve the problem.A guy wire to a tower makes a 67° angle with level ground. At a point 37 ft farther from the tower than the wire but on the same side of the base as the wire, the angle of elevation to the top of the pole is 32°. Find the wire length (to the nearest foot).
A. 39 ft B. 68 ft C. 34 ft D. 73 ft
Solve problems using a calculator.The Passive House Standard is a strict building code used as a state guideline for building energy-efficient homes. The total primary energy consumption of a building following this code must not be more than 19,020 Btu per square foot per year. What would this annual maximum be for a 4500-square-foot house?
A. 85,540,000 Btu per year B. 85,590,000 Btu per year C. 85,570,000 Btu per year D. 85,530,000 Btu per year
Solve the problem.Find the length of the arc intercepted by a central angle of 
in a circle of radius 
Round to two decimal places.
A. 0.81 cm B. 14.66 cm C. 7.33 cm D. 1.23 cm
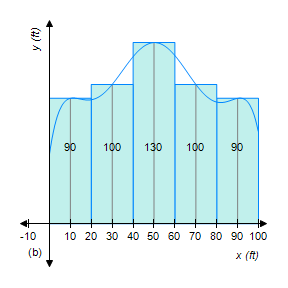
Figure (a) shows a vacant lot with a 100-ft frontage in a development. To estimate its area, we introduce a coordinate system so that the x-axis coincides with the edge of the straight road forming the lower boundary of the property, as shown in Figure (b). Then, thinking of the upper boundary of the property as the graph of a continuous function f over the interval [0, 100], we see that the problem is mathematically equivalent to that of finding the area under the graph of f on [0, 100]. To estimate the area of the lot using a Riemann sum, we divide the interval [0, 100] into five equal subintervals of length 20 ft. Then, using surveyor's equipment, we measure the distance from the midpoint of each of these subintervals to the upper boundary of the property. These measurements give the
values of f(x) at x = 10, 30, 50, 70, and 90. What is the approximate area of the lot?
?
?
?
__________ square feet
Fill in the blank(s) with the appropriate word(s).