Find the minimum or maximum value of f (as indicated) subject to the given constraint.Minimum of f(x, y) = x2 + y2 - xy,subject to x - y = 10
A. Maximum = 25 at (5, 5)
B. Maximum = 75 at (5, -5)
C. Maximum = 7 at (2, -1)
D. Maximum = 3 at (1, 2)
Answer: B
You might also like to view...
Find the gradient field of the function.f(x, y, z) = x10e5x + y7z5
A. ?f = (10 + 5x)x9e5xi + (x10e5x + 7y6z5)j + (x10e5x + 5y7z4)k B. ?f = (10 + 5x)x9e5xi + 7y6j + 5z4k C. ?f = (1 + x)x9e5xi + y6z5j + y7z4k D. ?f = (10 + 5x)x9e5xi + 7y6z5j + 5y7z4k
Identify the vertex, axis, domain, and range of the parabola.f(x) = (x - 5)2 + 6
A. vertex: (5, 6) axis: x = 5 domain: (-?, ?) range: [6, ?) B. vertex: (-5, 6) axis: x = -5 domain: (-?, ?) range: [6, ?) C. vertex: (-5, -6) axis: x = -5 domain: (-?, ?) range: [-6, ?) D. vertex: (5, -6) axis: x = 5 domain: (-?, ?) range: [-6, ?)
Perform the operation and give the answer as a fraction in lowest terms.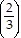
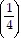
A. 
B.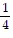 
C.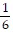 
D.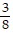 
Solve the problem.If u = 
, v = 
, and w = 
, evaluate (u + v) ? w.
A. -35 B. -37 C. -46 D. -42