Answer the question.Explain why the vertex of a parabola must lie on its axis.
What will be an ideal response?
The vertex of a parabola must lie on its axis because, by definition, the axis is a line that intersects the vertex, dividing the parabola into two equal parts. For the parabola defined by the following function:
the vertex is at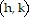 
and the vertical line x = h is the axis of the parabola. This vertical line includes all ordered pairs 
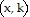and thus clearly includes 
.
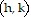You might also like to view...
Sketch a typical level surface for the function.f(x, y, z) = x - y2 - z2
A.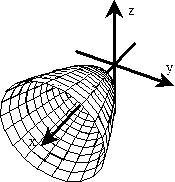 
B.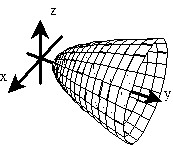 
C. 
D. 
Find the absolute value.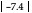
A. 0 B. -7.4 C. 7.4 D. ± 7.4
Find the domain and range of the inverse of the given function.f(x) =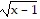 
A. Domain: [0, ?); range: [1, ?) B. Domain and range: all real numbers C. Domain: [1, ?); range: [0, ?) D. Domain: [1, ?); range: [1, ?)
Rewrite the expression as the sum and/or difference of logarithms, without using exponents. Simplify if possible.log2
A. 8 log2x + 7 log2y + log25 B. (8 log2x)(7 log2y) - log25 C. 8 log2x - 7 log2y - log25 D. 8 log2x + 7 log2y - log25