Solve the problem.On a sunny day, a flag pole and its shadow form the sides of a right triangle. If the hypotenuse is 40 m long and the shadow is 32 m, how tall is the flag pole?
A. 51 m
B. 24 m
C. 72 m
D. 64 m
Answer: B
You might also like to view...
Find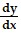 
by using logarithmic differentiation.y = (x3 + 1)2(x - 1)4x2
A.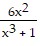 
+ 
+ 
B. (x3 + 1)2(x - 1)4x2(2ln(x3 + 1) + 4ln(x - 1) + 2ln x)
C. (x3 + 1)2(x - 1)4x2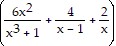
D. (x3 + 1)2(x - 1)4x2
Match the given equation with one of the graphs.y2 - 4x2 = 4
A. 
B. 
C.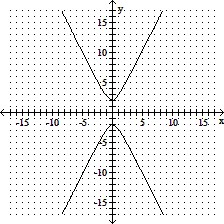 
D.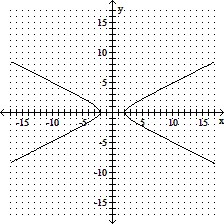 
Identify the function's local and absolute extreme values, if any, saying where they occur.f(x) = -x3+ 1.5x2 + 6x - 2
A. local maximum at x = 1; local minimum at x = -2 B. local maximum at x = -2; local minimum at x = 1 C. local maximum at x = 2; local minimum at x = -1 D. local maximum at x = -1; local minimum at x = 2
Find the product.(3y2 - 2y - 1)(y2 + 4y - 3)
A. 3y4 + 12y3 - 17y2 + 2y + 3 B. 3y4 + 10y3 - 17y2 + 2y + 3 C. 3y4 + 12y3 - 18y2 + 2y + 3 D. 3y4 + 10y3 - 18y2 + 2y + 3