Plot the point given in polar coordinates.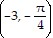
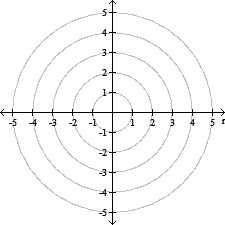
A. 
B. 
C. 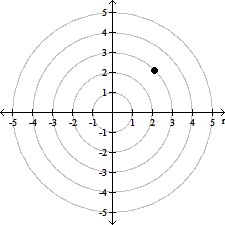
D. 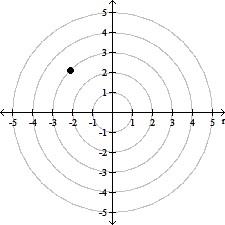
Answer: D
You might also like to view...
Solve the problem.Find the center of mass of the region of constant density bounded by the paraboloid  and the xy-plane.
and the xy-plane.
A.  = 0,
= 0,  = 0,
= 0,  =
= 
B.  = 0,
= 0,  = 0,
= 0,  =
= 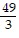
C.  = 0,
= 0, 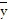 = 0,
= 0,  =
= 
D.  = 0,
= 0,  = 0,
= 0,  =
= 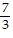
Use the rational zero theorem to find all possible rational zeros for the polynomial function.P(x) = -2x4 + 3x3 + 2x2 + 18
A. ±1, ± 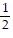 , ±2, ±3, ±
, ±2, ±3, ±  , ±6, ±9, ±
, ±6, ±9, ± 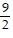 , ±18
, ±18
B. ±1, ±2, ±3, ±6, ±9, ±18
C. ±1, ±2, ±  , ±
, ± 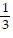 , ±
, ± 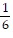 , ±
, ± 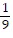 , ±
, ± 
D. ±1, ± 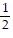 , ±2, ±3, ±6, ±9, ±18
, ±2, ±3, ±6, ±9, ±18
Solve.The outline of a certain planet can be considered a circle with a radius of approximately 2000 miles. Thus, if the center of this planet is located at (0, 0), then an equation for this circle is 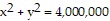 . Suppose an incoming meteor is approaching the planet in a hyperbolic path. The equation describing the meteor's path is
. Suppose an incoming meteor is approaching the planet in a hyperbolic path. The equation describing the meteor's path is 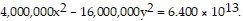 Will the meteor strike the planet? If so, locate the point (x, y) where the meteor will strike. Assume that x and y are both positive, and round to three significant digits.
Will the meteor strike the planet? If so, locate the point (x, y) where the meteor will strike. Assume that x and y are both positive, and round to three significant digits.
A. Yes, at the point (x, y) ? (3270, 2000) B. Yes, at the point (x, y) ? (2530, 2000) C. Yes, at the point (x, y) ? (2310, 3060) D. No, the meteor will not strike the planet.
Evaluate the expression without using a calculator.-2431/5
A. -27 B. 81 C. 243 D. -3