Solve the problem. Round to tenths where necessary.A baseball team is trying to determine what price to charge for tickets. At a price of $10 per ticket, it averages 45,000 people per game. For every increase of $1, it loses 5,000 people. Every person at the game spends an average of $5 on concessions. What price per ticket should be charged in order to maximize revenue?
A. $4.00
B. $7.00
C. $3.00
D. $13.00
Answer: B
You might also like to view...
Find T, N, and B for the given space curve.r(t) = (8t sin t + 8cos t)i + (8t cos t - 8 sin t)j - 6k
A. T = (-cos t)i - (sin t)j; N = (sin t)i - (cos t)j; B = -k B. T = (-cos t)i - (sin t)j; N = sin(t)i - (cos t)j; B = 6k C. T = (-cos t)i - (sin t)j; N = (sin t)i - (cos t)j; B = k D. T = (-cos t)i + (sin t)j; N = (sin t)i + (cos t)j; B = -k
Sketch the graph of the given equation. Begin by sketching the traces in the coordinate planes.x2 + y2 + z2 = 4
What will be an ideal response?
Solve the problem.A company found that the cost (in $) of producing x lb of a solvent is given by C(x) = 2x3 - 19x2 + 110x - 492. Find x for C(x) = $33.
A. 10 B. 9 C. 12 D. 7
Find the integral.
A. 
x2e2x - xe2x + 
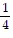e2x + C
B.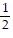 
x2e2x - 
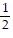xe2x + 
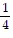e2x + C
C.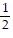 
x2e2x - 
xe2x +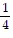 
e2x + C
D.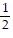 
x2e2x - 
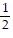xe2x + C