Find the vertex, focus, and directrix of the parabola with the given equation.y2 + 12y = 8x - 12
A. vertex: (-3, -6)
focus: (-5, -6)
directrix: x = -1
B. vertex: (-3, -6)
focus: (-3, -4)
directrix: y = -8
C. vertex: (-3, -6)
focus: (-1, -6)
directrix: x = -5
D. vertex: (-3, -6)
focus: (-3, -8)
directrix: y = -4
Answer: C
You might also like to view...
Find the absolute maxima and minima of the function on the given domain.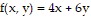
on the closed triangular region with vertices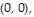 
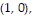and 
A. Absolute maximum: 4 at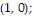 
absolute minimum: 0 at 
B. Absolute maximum: 10 at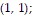 
absolute minimum: 4 at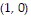 
C. Absolute maximum: 6 at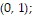 
absolute minimum: 0 at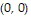 
D. Absolute maximum: 6 at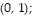 
absolute minimum: 4 at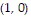 
Find the derivative.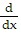
A. 10x9 cos (x5) B. cos (x5) - 1 C. sin (x5) D. cos (x5)
Use implicit differentiation to find d2y/dx2.2y - x + xy = 2
A.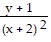 
B.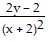 
C.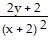 
D.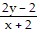 
Find the value of c for which the expression is a perfect-square trinomial. Then factor the perfect-square trinomial.x2 + 5x + c
A. 10; (x + 5)2
B.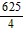 
; 
2
C. - 
; 
2
D. 
; 
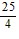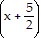2