Solve the problem.If two sound sources at the same volume are equidistant from a microphone, the pressure on the microphone is given by p = a cos ?1t + a cos ?2twhere a, ?1, ?2 are constants and t is time. Write p as a product of cosine functions.
What will be an ideal response?
p = 2a cos (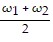
t) cos (
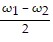t)
You might also like to view...
Decide whether or not the given equation is an identity.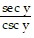
= tan y
A. Yes B. No
Provide an appropriate response.Explain why f(x) = 2x is an exponential function but f(x) = x2 is not.
What will be an ideal response?
Determine whether the graph is that of a function. If it is, use the graph to find its domain and range, the intercepts, if any, and any symmetry with respect to the x-axis, the y-axis, or the origin.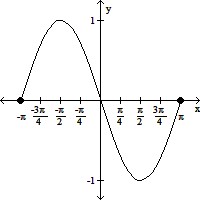
A. function domain: {x|-1 ? x ? 1} range: {y|-? ? y ? ?} intercepts: (-?, 0), (0, 0), (?, 0) symmetry: none B. function domain: {x|-? ? x ? ?} range: {y|-1 ? y ? 1} intercepts: (-?, 0), (0, 0), (?, 0) symmetry: origin C. function domain: all real numbers range: {y|-1 ? y ? 1} intercepts: (-?, 0), (0, 0), (?, 0) symmetry: origin D. not a function
Draw two arrays to represent the product.2 times 3
A.
 ** |  |
B.
 ** |  |
C.
 ** |  |
D.
| * *? | * * |